
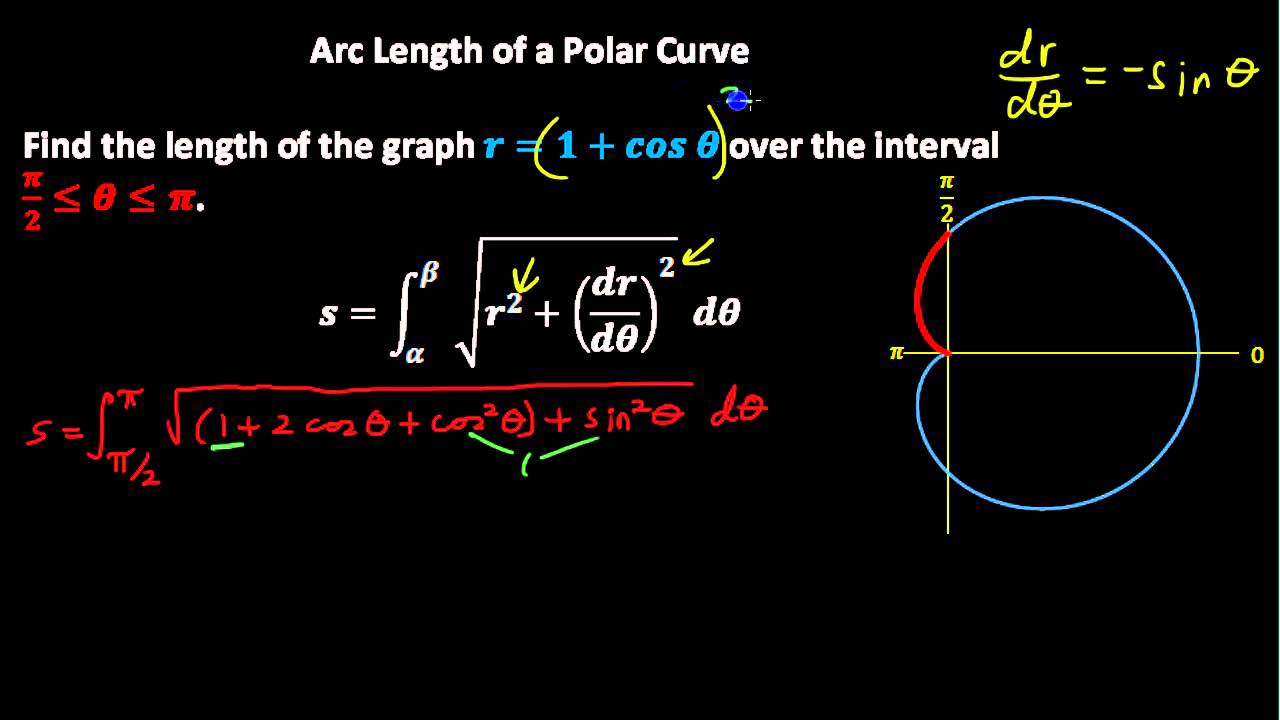

Lets first begin by finding a general formula. What you have to do is substitute in the integral the values of the derivative and the square of \rho: The following problems involve the computation of arc length of differentiable functions on closed intervals. The function and its derivative must both be continuous on. To do so, let’s multiply the 2 with the parenthesis:Īnd now we proceed to derive, derivative of a constant is equal to zero and derivative of cosine is equal to -\sin: The length of an arc along a portion of a curve is another application of the definite integral. Because these to square roots do not simplify any further, you have to express the arc length for this function and these bounds as 257 17. In this case, you get the square root of 257. In mathematics, the arc length of a curve in the plane or in space is the length of a curve from a fixed reference point on the curve to its endpoints. As it says in the formula, we need to calculate the derivative of \rho. Next, use the same formula and plug 4 into x. Calculate the circumference of the circle using the arclength formula by using the following steps: (a) Show that the Cartesian equation of the unit circle is.


 0 kommentar(er)
0 kommentar(er)
